for graphically finding the roots of polynomials.
Draw the coefficients of a polynomial as lines with length ![]() , turning
, turning ![]() to the left with each one. The slope of lines drawn from one end to the other, bouncing off the sides, give the roots. Very snookery, except the balls bounce off at
to the left with each one. The slope of lines drawn from one end to the other, bouncing off the sides, give the roots. Very snookery, except the balls bounce off at ![]() , not at equal angle. It works because, remarkably, the expressions for each angle as the ball bounces off the sides are the same as the partial Horner forms of the polynomial. So ‘ball goes in the pocket’ = ‘its slope is a root of the equation’.
, not at equal angle. It works because, remarkably, the expressions for each angle as the ball bounces off the sides are the same as the partial Horner forms of the polynomial. So ‘ball goes in the pocket’ = ‘its slope is a root of the equation’.
+++++I’d never heard of this until recently, apparently it used to be very well-known, 100+ years ago, but is obscure now. Can’t make money from it, I guess.
e.g. ![]() : in this example, the “balls'” paths initially have slopes of -1, -2, -3, -4.
: in this example, the “balls'” paths initially have slopes of -1, -2, -3, -4.

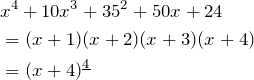
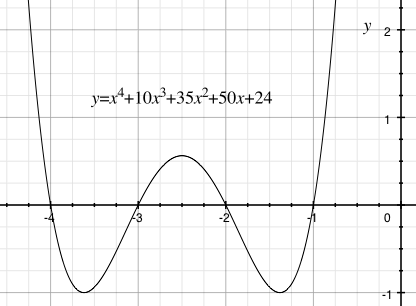
The Carlyle circle method, apparently invented by the essayist/historian Thomas Carlyle in his youth:
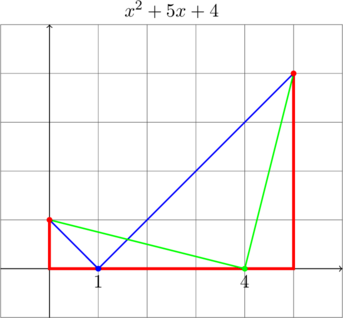
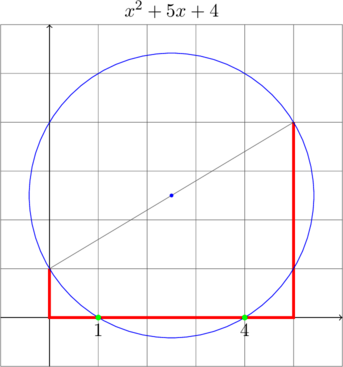
references
Phillips Bradford’s Lill’s method applets
Bradford’s page on Lill’s method, including finding imaginary roots
M. Riaz, Geometrical Solution of Algebraic Equations, 1962
Bixby’s 1879 pamphlet
Magnus Holm’s cubic Lill’s method applet
Geometrical Construction of Roots of Quadratic Equation at cut-the-knot
Tom Hull talk on Origami and Constructible Numbers, includes explanation of why Lill’s Method works and can be done in origami
Carlyle Circle at Wolfram mathworld
Geometrical and Graphical Solutions of Quadratic Equations by John Hornsby, Jr., The College Mathematics Journal, Vol. 21, No. 5 (Nov., 1990) (pdf)