I got the idea from V.I. Arnold’s Real Algebraic Geometry, where he multiplies lines together (e.g. and ) then increases the RHS above to create disconnected shapes. Can make pretty much any shape that way. So immediately I thought of chess boards.
Well, this was my first effort in the area:

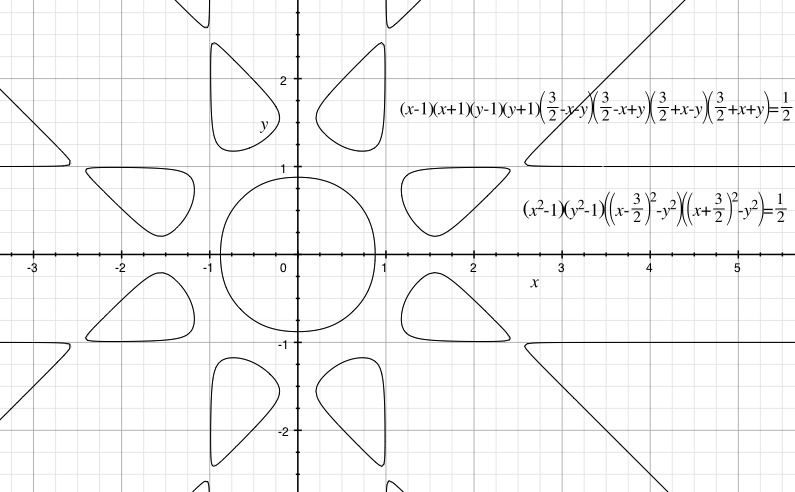
Then chess boards.
gives the “traditional” square grid; increasing the RHS from makes the squares gradually circular, eventually the central ones disappear. (I used Mac Grapher for these pictures; it has problems drawing it accurately, even on highest settings.)




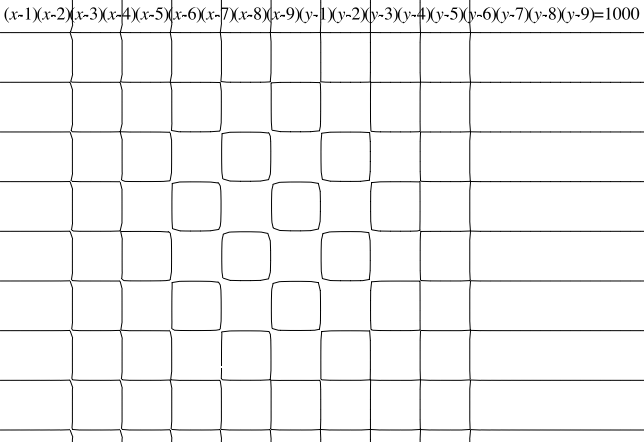
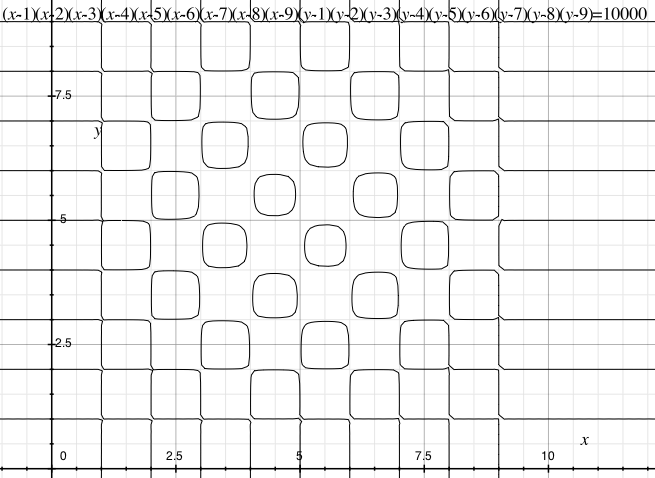
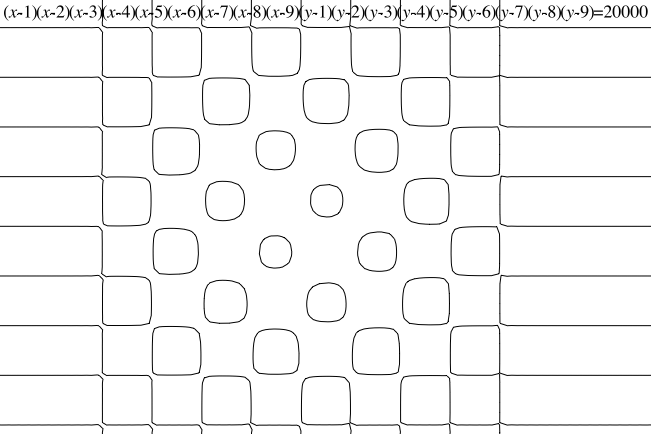
10000 (although I haven’t tried to play on it yet!) seems a good balance between craziness and playability:

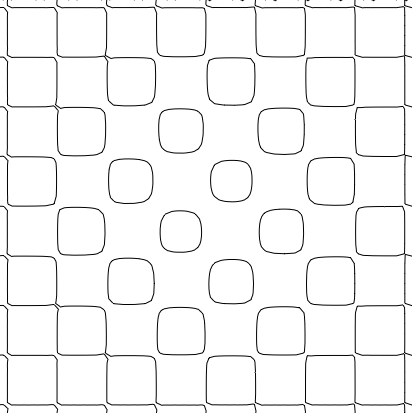
Or in coloured form:

Well that looks awful; I turned the original into b&w which messed up the smoothing. Will do a better version. But you get the idea.

Another try with some border lines added; I thought it might slow down the (possibly excessive) circlification in the centre:

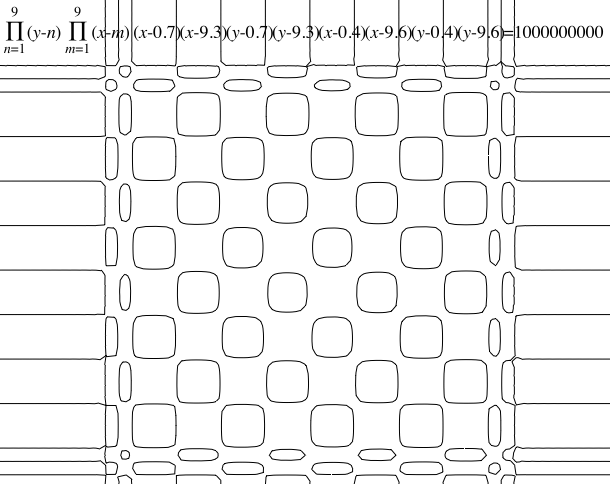
I would have made them closer together, but Grapher can’t draw that at all.

Next: Why not just use circles. Or those squarish circles with ?

references

V.I. Arnold – Real Algebraic Geometry, p.41